« Neuere Beiträge Seite 2 von 9 Ältere Beiträge »
Bilder vom Tag des offenen HackerspaceKeiner hackt feiner! Besucht uns am internationalen Tag des offenen Hackerspaces!
Safer Internet Day 2023
Hnefatafl
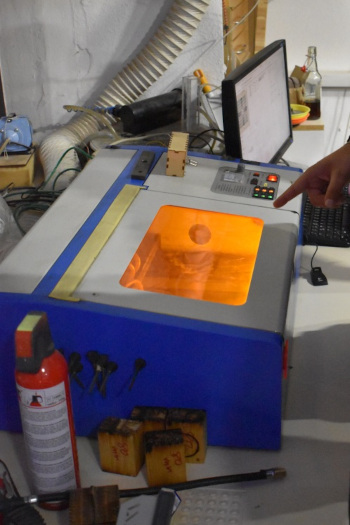
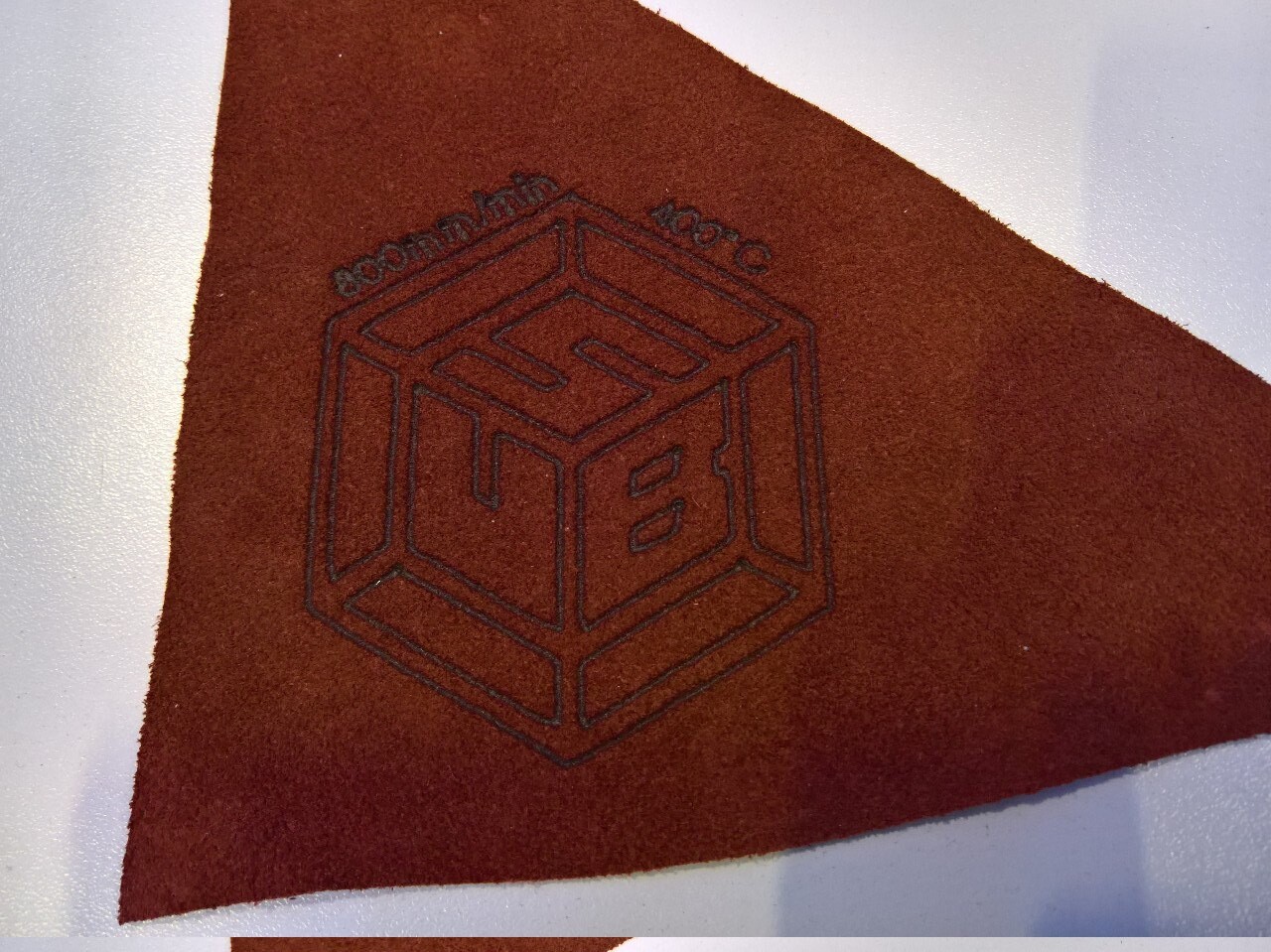
Ledergravur
Dodekaeder beim Paderborner Martinslauf
Einladung zum Tag des offnen Hackerspaces